Example 2

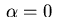We choose $\alpha=0$ in Example 1 and expect to obtain a bang-bang control. The adjoint equation agrees with that in (4.1). In view of $\,\alpha=0\,$ the control law (2.22) yields

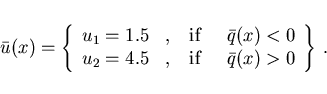$$\hspace*{-8mm} \bar{u}(x) = \left \{ \begin{array}{llllll} u... ... \,\mbox{if} & \;\; \bar{q}(x) > 0 \end{array}\right \} \,. \;$$ (4.3)

Table 2: Information on solution of Example 2

N+1	it	CPU	Acc	$F(\bar{y})$
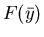50	30	112	8	.0521138
100	37	2169	8	.0564474
200	45	67769	8	.0596968

Figure 3 shows that the optimal control is indeed bang-bang and does not exhibit singular parts. The optimal state and adjoint variables are displayed in Figure 4. Both figures allow to verify precisely the switching conditions of the control law (4.3).