


Next: Boundary control problem
Up: paper87
Previous: Introduction
Necessary conditions for elliptic control problems
with control and state constraints
Let
 be a bounded domain
with piecewise smooth boundary
be a bounded domain
with piecewise smooth boundary
 .
The derivative in the direction of the outward unit normal
.
The derivative in the direction of the outward unit normal 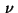 of
of
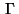 will be denoted by
will be denoted by
 .
Suppose that the boundary is partioned as
.
Suppose that the boundary is partioned as
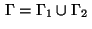 with disjoints sets
with disjoints sets
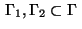 that consist of finitely
many connected components.
that consist of finitely
many connected components.
Subsections
Hans D. Mittelmann
2000-12-09